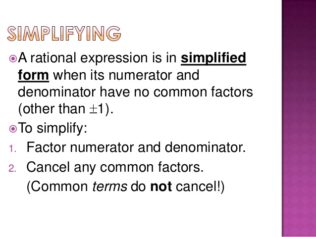
Overview
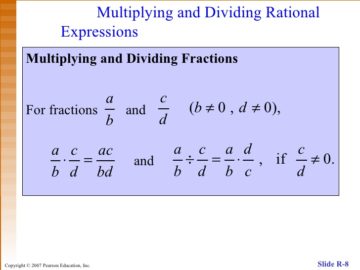
The process of multiplying and dividing rational expressions is similar to multiplying and dividing rational numbers. In order to multiply, first multiply the numerators, then the denominators, and then simplify the expression. Division is the inverse of multiplication, so multiply by the reciprocal (or inverse) of the divisor, and then simplify the expression.
Multiplying Rational Numbers
Rational numbers are also known as fractions. The fraction ¾ is a rational number, as is the fraction 3/5. The numerator 3 is in a ratio to the denominator 4. To multiply ¾ by 3/5, multiply the numerators 3·3, and then multiply the denominators 4·5, to result in the new fraction or ratio of 9/20. The fraction 9/20 is in the simplest form, because there are no factors common to both 9 and 20 except for 1. Suppose one ratio were a/9 and another were 7/10. The process of multiplication would be similar, so that a/9 times 7/10 would equal 7a/90.
Multiplying Rational Expressions
Suppose the problem were (5a3)/4 times 2/ (5a). The numerator is 5a3·2 or 10a3, and the denominator is 4 ·5a or 20a. The new expression, (10a3)/20a can be simplified to ½ ·a2 or a2/2. If the problem were 4/ (5x2) ·(x-2)/ (2x3), the new numerator would be 4(x-2) and the new denominator would be (5x2) · (2x3) or 10x5. The new expression is then [4(x-2)]/ (10x5), which is not in simplest form. The fraction 4/10 can be simplified to 2/5, so the expression in simplest form is [2(x-2)]/ (5x5)].
Dividing Rational Numbers
Remember that dividing rational numbers is the same as multiplying by the reciprocal of the divisor, so that 4/5 ÷2/3 is the same as 4/5 ·3/2, so that 4·3 equals 12 and 5·2 equals 10. Since 2 is a common factor in both the numerator and denominator, the fraction in simplest terms is 6/5. There are no common factors to both 6 and 5 except for 1 so the fraction is in simplest terms.
Dividing Rational Expressions
Similar to dividing rational numbers, when dividing rational expressions, also multiply by the reciprocal of the divisor. Therefore (8n5)/3 ÷ (2n2)/9 becomes (8n5)/3 ·9/ (2n2). Multiply [(8n5) · 9], the numerator and [3· (2n2)]. However, the resulting expression (72n5)/ (6n2) is not in simplest terms. First, factor out the common coefficients, so that 12 is left. (The number 72 divided by 6 equals 12.) Next factor out the common variables, so that n5/n2 equals n3. The quotient in simplest form is 12n3. Likewise, suppose the problem were [(4m-8)/5] ÷ [(m-2)/10]. The new expression would then be [(4m-8)/5] · [10/ (m-2)]. The new numerator can be factored as [4(m-2)]10, and the new denominator can be factored as 5(m-2). Since (m-2) is a common factor for both the numerator and the denominator, (m-2)/(m-2) equals 1 and cancels out, leaving 4(10)/5, or 8.
Interested in math tutoring services? Learn more about how we are assisting thousands of students each academic year.
SchoolTutoring Academy is the premier educational services company for K-12 and college students. To learn more about how we help parents and students in Charlottetown, PE, Canada: visit: Tutoring in Charlottetown, PE