Triangles are an important shape that come up all over the place in life, which is why trigonometry is one of the most important fields of mathematics. Pythagorean theorem is incredibly important for many different real life applications, such as architecture, construction, navigation, and surveying. Pythagorean Theorem allows us to calculate the side lengths of right-angled triangles using the formula:
c2 = a2 + b2
In a right triangle ( a triangle containing a 90o angle), c represents the hypotenuse, which is the longest side of the triangle. The hypotenuse is always located directly across from the 90 degree angle. a and b are the other two side lengths, and it doesn’t really matter which one you label b and which one you label a, as long as you keep track of how you decided to label them.
If you know every side length other than the hypotenuse, you can rearrange the formula to be
c = √(a2 + b2)
If you know the hypotenuse and one other side length, you can rearrange the formula as:
a = √(c2 – b2)
or
b = √(c2 – a2)
Example
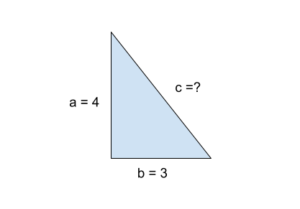
In this case a = 4, b=3, and we want to solve for c.
c= √(a2 + b2)
c = √(42 + 32)
c = √(16 + 9)
c = √(25)
c = 5
Word Problem example
The bottom of a ladder must be placed 3 feet from a wall. The ladder is 12 feet long. How far above the ground does the ladder touch the wall?
To solve word problems, the easiest method is to draw a diagram.

Now that we have a diagram of the information the problem is giving us, it is much easier to see what we have to do to solve it. The ladder, ground, and wall form a right-angle triangle, so we can use the Pythagorean theorem to solve for the missing side length in the triangle they form.
The hypotenuse, the longest side, is the ladder, which is 12 feet, and the other length we know is 3 feet which we can label as side a.
Taking our rearranged formula we know that:
c2 = a2 + b2
b2 = c2 – a2
b = √(c2 – a2
b = √(122 – 32)
b = √(144 – 9)
b = √(135)
b = 11.6 ft
Therefore, the ladder touches the wall 11.6 feet above the ground.
SchoolTutoring Academy is the premier educational services company for K-12 and college students. We offer tutoring programs for students in K-12, AP classes, and college. To learn more about how we help parents and students in Jackson, Missouri visit: Tutoring in Jackson, Missouri.




