Overview
Linear equations can be solved by isolating the variable on one side of the equals sign. This can be done by using the addition and multiplication properties, whether the variable is on one side of the equals sign or there is a variable on both sides of the equals sign.
Equations with the Variable on One Side of the Equals Sign
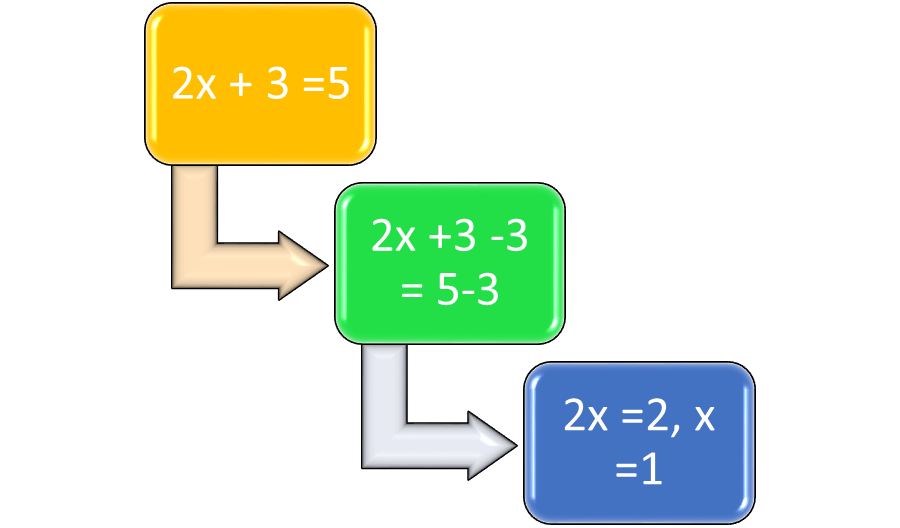
The goal when solving an equation is to isolate the variable on one side of the equation. That way, the constants are on the other side of the equals sign and the variable is on the other. The variable can be isolated by using the properties of addition, multiplication, or the distributive property of real numbers. Suppose the equation to be solved is 2x + 4 = 20. The variable can be isolated by using the property of addition on both sides of the equation, as 2x + 4 – 4 = 20 – 4. Then 2x = 16. Both sides of the equation can then be divided by 2 so (2x)/2 = 16/2, or x = 8. The solution can be checked by the original equation: 2∙8 + 4 = 20, which is a true statement.
Figure 1: Steps for solving equations.
Equations Containing Like Terms or Parentheses
Like terms or parentheses should be eliminated before isolating the variable on one side of the equation. Suppose the equation were 42x + 3 + 2x + 5 – 8 = 88. The first step would be to combine the like terms using the commutative property, so that 42x + 2x + 3 + 5 – 8 = 88, or 44x + 0 = 88. The variable is easy to isolate as 0 is the identity for addition and can be eliminated, so that 44x = 88. Dividing both sides by 2, using the multiplication property, (44x)/44 = 88/44, or x = 2. Checking the solution, 84 + 3 + 4 + 5 -8 = 88. Suppose the equation were 3y – (2y + 5) = 7. The parentheses can be eliminated first as 3y -2y -5 = 7. Combining like terms, y – 5 = 7 or y = 12.
Solving Linear Equations with the Variable on Both Sides of the Equals Sign
Solving linear equations when the variable on both sides of the equals sign is similar to solving a linear equation when the variable is on one side of the equals sign. The goal is still to isolate the variable on one side of the equation. Suppose the equation is 5x + 11 = 2x + 20. The variables can be isolated on one side or the other. If the variables are to be located on the left side, 5x – 2x + 11 = 2x – 2x + 20. Combining the like terms in variables, 3x + 11 = 20. Then, 3x = 20 – 11, or 3x = 9. Dividing both sides by 3, (3x)/3 = 9/3, or x = 3. If the variable is to be isolated on the right side, 5x -5x + 11 = 2x -5x + 20. Then, 11 = -3x + 20, 11 – 20 = -3x or -9 =-3x, (-3x)/3 = -9. Since a negative times a positive is a negative, x = 3.
Figure 2: Solving equations with the variable on both sides of the equals sign.
Do the Linear Equations Have a Solution?
Some linear equations with the variables on both sides have more than one solution. Suppose the equation to be solved is 6x – 3 = 2(3x – 1). Eliminating the parentheses, 6x -3 = 6x -3. Since the equations on both sides of the equals sign are the same equation, any solution for x will be a correct solution. If x = 0, -3 = -3. If x = 1, then 3 = 3. If x = 2, then 9 = 9, and so on. The solution for x is all real numbers. In contrast, some linear equations with the variable on both sides have no solutions. Suppose the equation were 4x + 9 = 2(2x + 5). Eliminating the parentheses, 4x + 9 = 4x + 10. Isolating the variable on one side, 4x -4x + 9 = 4x – 4x + 10 or 9 = 10. That is a nonsense statement, and means that the problem has no solution.
Figure 3: An equation with no solution.
Interested in algebra tutoring services? Learn more about how we are assisting thousands of students each academic year.
SchoolTutoring Academy is the premier educational services company for K-12 and college students. We offer tutoring programs for students in K-12, AP classes, and college. To learn more about how we help parents and students in Macon, GA: visit Tutoring in Macon, GA