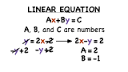Overview
Linear equations can be graphed by using intercepts or by changing the equation to standard form to make it easier to graph. Horizontal and vertical lines have special relationships in terms of the standard form. The slope of a line is a ratio that describes the steepness and direction of the line.
Graphing by Using Intercepts
Often, the easiest way to graph a line on the Cartesian coordinate system is by using the points where the line crosses the x axis and the y axis. The x-intercept of a line is the x coordinate of the point where the line intercepts the x axis. The y-intercept of a line is the y coordinate of the point where the line intercepts the y axis. For example, in the graph below, the x coordinate of the x intercept is -4 and the y coordinate of the y-intercept is 6. Notice that the y coordinate of the x intercept is 0 and the x coordinate of the y intercept is 0.
Standard Form of Linear Equations
The standard form of a linear equation is the form ax +by = c, where a, b, and c are constants and either a or b can be zero, but not both of them in the same equation. An equation such as 3x +4y = 12 is already in standard form, because 3, 4, and 12 are all constants. An equation such as 2y = 3x – 6 is not in standard form, but it can easily be put into standard form as 2y – 3x = -6.
Horizontal and Vertical Lines
The equations of horizontal lines have a special relationship to standard form. Suppose the equation is y =4. How can that be put in standard form? The coefficient of x is 0, or 0x +y = 4. Any value of the x coefficient is possible as long as y equals 4. Any ordered pair will be a solution to the equation y=4 as long as y is 4, so (-32, 4) (-9, 4) (0, 4) and so on are all points along that line. Similarly, suppose the equation is x =-5. This time, any value of the y coefficient is possible as long as x equals -5.
Slope
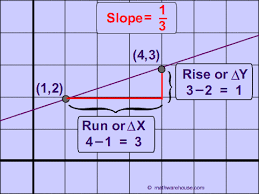
The slope of a line is a ratio that describes the steepness and direction of the line. It is defined as the change in the y coordinates or the rise, divided by the change in the x coordinates or the run. Suppose a line contains the points (1, 2) and (4, 3). The change in the y coordinates (Δy) can be expressed as 3-2 or 1, and the change in the x coordinates (Δx) can be expressed as 4-1 or 3. Rise/run is 1/3.
Interested in math tutoring services? Learn more about how we are assisting thousands of students each academic year.
SchoolTutoring Academy is the premier educational services company for K-12 and college students. We offer tutoring programs for students in K-12, AP classes, and college. To learn more about how we help parents and students in Londonderry, NH: visit: Tutoring in Londonderry, NH