Overview
Similar to systems of linear equations, systems of linear inequalities can be solved graphically, by using substitution, or by using elimination. If the systems are solved graphically, or represented on the coordinate plane, the location of the solutions will depend upon the nature of the inequality.
Review of Linear Inequalities
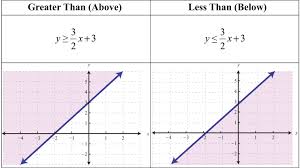
The solution set for a linear equation appears on the coordinate plane as a line that contains all the ordered pairs that make the equation true. The solution set for an inequality appears as a section of the coordinate plane. Suppose that the linear equation is x=y. The coordinates of the line for the solution set will pass through (0, 0), (1, 1), (2, 2) and so on. If the inequality is x > y, the solution set for x = y will be represented by a dotted line, and then all the points that are above that line will be part of that solution set. If the inequality is x≥ y, the solution set will include the points on the line x = y, as well as all the points above that line. If the inequality is x< y, all the points will be below the dotted line for x=y, and if the inequality is x≤ y, the solution set will contain the line for x =y also.
Figure 1: Review of linear inequalities
Systems of Linear Inequalities
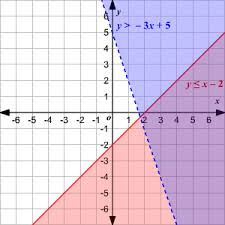
When the linear inequalities for a system of expressions are shown graphically, the solution set for the system contains the points that fulfil both expressions. Suppose that the inequality y> -3x + 5 is graphed and shaded in blue, and the inequality y≤ x – 2 is graphed and shaded in red. Note that the blue inequality is shaded above the dotted line, as there is no equals sign, and the red inequality is shaded below a solid line, because there is an equals sign. The points that solve the system of inequalities are in purple, because they satisfy both the red and blue conditions.
Figure 2: The solution set of a system of inequalities contains points that satisfy both conditions.
Solving Inequalities by Substitution
Similar to equations, inequalities can be solved using the substitution method. Suppose that a pair of inequalities were represented by y > 2x – 1 and x = 3. In that case y > 6 – 1 or y >5, when x = 3.
Solving Systems by Elimination
Also, systems of inequalities can be solved using the elimination method. Suppose that the system of inequalities were 2x + 3y >4 and -2x + y ≥ 6. The x variable is cancelled out to leave the inequality 3y + y ≥ 10, or 4y ≥ 10, or 2y≥ 5 so that y≥ 5/2. If -2x + 5/2 is ≥ 6, then x ≥ -1.75, and y is 2.5.
Figure 3: Example of using elimination to solve a system of equations.
Interested in algebra tutoring services? Learn more about how we are assisting thousands of students each academic year.
SchoolTutoring Academy is the premier educational services company for K-12 and college students. We offer tutoring programs for students in K-12, AP classes, and college. To learn more about how we help parents and students in Bountiful, UT: visit Tutoring in Bountiful, UT