Overview
Using infinitesimal quantities to approximate measurement of any item is an ancient way to determine the size and shape of irregular objects. Although it was very controversial in the 17th and 18th century Europe, the practical aspects of using infinitesimal quantities in calculations led to advances in science, engineering, and technology, along with the development of calculus.
Geometric Infinitesimals
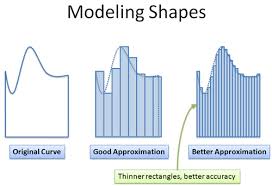
Greek mathematicians such as Archimedes used the smallest possible indivisibles to find areas of solids. In the 17th century, the astronomer and mathematician Johannes Kepler looked at a different way to compute the area of a circle or curved figure. Suppose a circle or curve were made up of infinitely many polygons, like thinner and thinner slices of pie. The sum of all those slices would equal the area of the circle or curved figure, even though the area of one slice was infinitesimal.
The Controversy
Slicing a figure into infinitely many thin fragments was very attractive to many mathematicians and scientists, because it solved a number of practical problems. However, many philosophers hated the idea. Using infinitesimals in mathematical calculations was banned in Rome in the 1600’s, and denounced from pulpits and in books.
Practical Use of Infinitesimals
Infinitesimals are close to zero and retain properties such as angles or slopes. Before calculus, mathematicians, scientists, and engineers could use infinitesimal quantities in calculations such as finding the area under a curve, or approximating the rate of change. Suppose that a large tank holds 1000 gallons of water. The instantaneous rate that water drains from the tank can be calculated using infinitesimal approximations.
Leibniz, Newton, and Calculus
Infinitely small building blocks (such as 1/∞) add up to something if enough of them are used. The mathematician and philosopher Gottfried Leibniz used those and other mathematical observations to promote a new system of mathematics to calculate areas under curves called calculus. At the same time, the more famous Sir Isaac Newton developed a similar system of calculus, to be applied to many aspects of mathematical physics. Although it was very controversial in the 1700s, both Leibniz and Newton made independent contributions to a new method using mathematics to describe the natural world.
Interested in pre-calculus tutoring services? Learn more about how we are assisting thousands of students each academic year.
SchoolTutoring Academy is the premier educational services company for K-12 and college students. We offer tutoring programs for students in K-12, AP classes, and college. To learn more about how we help parents and students in Louisville, KY: visit: Tutoring in Louisville, KY