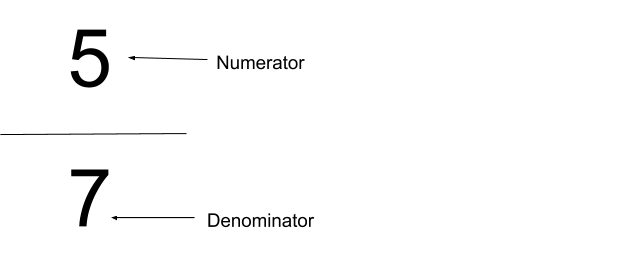
Derivative: an expression that represents the rate of change of a function. At this level, it is usually denoted as y’ or f’(x) (read y prime and f prime at x respectively)
Derivatives are often the first concept that is taught in Calculus. Understanding how to find the derivative of a function is essential to your success in the course. The article below provides details on the five basic methods used.
-
Power Rule
The power rule provides a method for taking the derivative of any polynomial function.
When doing so, we consider each algebraic term separately, applying the following rule:
The derivative of y = ax^b is y’ = a*bx^(b-1)
Keep in mind that terms without variables are constant so their rate of change is zero and therefore their derivative is defined as 0.
Example: The derivative of 3x^3 + 6x^2 – 3x + 10 is 9x^2 + 12x – 3
Pro Tip: Remember that irrational numbers represented by symbols, like ℼ and e, are still constants and therefore their derivative is 0.
-
Product Rule
The product rule provides a method for taking the derivative of a function created by the multiplication of two functions. We use the following formula:
The derivative of f(x) = g(x)h(x) becomes f’(x) = g(x)h’(x) + g’(x)h(x)
where g’(x) and h’(x) are the derivatives of g(x) & h(x)
Example: The derivative of (2x^2 – 1)(x^4 – 2) is 4x(x^4 – 2) + (2x^2 – 1)(4x^3)
which can be simplified to 12x^5 – 4x^3 – 8x
-
Derivatives of Basic Trig Functions
The three basic trigonometric functions are sin(x), cos(x), and tan(x). The best way to handle the derivatives of these functions is to memorize them.
| Function | Derivative |
| sin(x) | cos(x) |
| cos(x) | –sin(x) |
| tan(x) | sec^2(x) |
-
Derivative of Exponential Functions
There is a handy formula for taking the derivative of exponential functions.
The derivative of A^x is ln(A) * A^x
Example: The derivative of 2^x is ln(2) * 2^x
-
The Chain Rule
The chain rule is the most complex, but it is also the one that allows for the most flexibility. It provides a method for taking the derivative of a composite function. We use the following formula:
The derivative of f(x) = g(h(x)) is f’(x) = h’(x)g’(h(x))
Example: The derivative of sin(x^2) is 2x * cos(x^2)
The derivative of cos^2(x) is -sin(x) * 2cos(x)
which simplifies to -sin(2x)
using the trig identity 2cos(x)sin(x) = sin(2x)
Interested in math tutoring services? Learn more about how we are assisting thousands of students each academic year.
SchoolTutoring Academy is the premier educational services company for K-12 and college students. We offer tutoring programs for students in K-12, AP classes, and college. To learn more about how we help parents and students in Chattanooga, Tennessee: visit: Tutoring in Chattanooga, Tennessee.